It’s been cold here in the Midwest. In ten days in La Crosse, WI, where I am, the average low was -13.7F, average high, 4.3, and the average of averages over the ten days was -4.7F. We smashed one daily record. There have been no all-time lows, but this is a grind.
In the middle of this grind,[1] I woke up one morning thinking about greenhouse gases, GHGs. There is very little GHG in this weather because the dominant GHG is water vapor, and below-zero air has almost no capacity to hold water. Then I got to thinking; this is a good excuse to write a tribute to one of my great teachers that moved me along in the direction of where I am today; and a good promotion for STEM[2] for kids.
My journey for STEM started when I was a little kid using math flashcards. My parents, neither of whom had post-high-school educations, always pushed us hard into math and science courses.
My junior ha school science teacher, Craig Kroger, as I recall, fascinated me with numbers and taught me the fast and easy way of unit cancellation (examples below). I learned nearly everything I needed to know about GHGs before global warming was a thing.
I would wager that most readers don’t know what a ton of carbon dioxide represents. We’re used to weighing things in the presence of ambient air. If the scale says you weigh 120 lbs, you actually weigh 120 lbs plus the mass of the air you’re displacing, which is negligible compared to 120 lbs. But ambient air, which is about 21% oxygen (O2), 78% nitrogen (N2), and 1% of everything else, including about 0.04% CO2, has mass.
Moles, Not of the Burrowing Type
Stick with me! A mole of a substance is 6.022 x 10^23 = 602,200,000,000,000,000,000,000 molecules of the substance. Say hello to big numbers. That number of molecules has a mass equal to the molecular weight in grams of the substance. How do I know this? Junior ha! That’s what good teaching does!
A chap named Amedeo Avogadro determined this number (Avogadro’s number), AND that gases of equal numbers of molecules, a mole, at the same temperature and pressure take up the same volume. These are things I’ve never forgotten.
Brain Blowing Numbers
Let’s give Avogadro’s number perspective. The United States used 101 quadrillion Btus (quads) of energy in 2018. A Btu is a tiny measure of energy. In this coldest weather, it takes a million of them in the heating value of natural gas to keep my house warm for one day.
So get this: The number of molecules of any gas, say CO2 or O2, that would fit in a shoebox is 6 million times greater than the 101 Quads of energy used in the US in a year. If that doesn’t blow your mind, math, physics, or engineering are probably not for you. That is pure cool to me.
Now consider a periodic table of the elements, some of which I also remember, and you look up the molecular weights of various constituents of air and natural gas:
- Hydrogen: 1
- Carbon: 12
- Nitrogen: 14
- Oxygen: 16
These are elemental weights, meaning the weight of one atom. The molecular weight of H2O is 18 (2×1 +16 = 18). The molecular weight of CO2 is 44. The molecular weight of methane (CH4, aka natural gas) is 16. So a mole of any of these things is equal to the molecular weight, in grams.
What’s a gram? A cubic centimeter of water weighs about 1 gram. One thousand grams (a kilogram) is about the same as 2.2 lbs.
GHG Calculation Example
Let’s use natural gas because it’s easy. When organics[3] burn, they break apart and rearrange themselves to form CO2 and H2O, and of course, release heat.
The Energy Information Administration says burning a million Btu of natural gas produces 117 lbs of CO2. So this could be a junior high test question: How many lbs of CO2 are produced by burning 1 million Btu of natural gas? Closed book, calculator, No. 2 pencil, and paper only.
Here is the chemical reaction of burning a mole of CH4 with two moles of air:
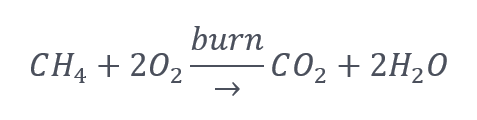 Why two moles of air per mole of natural gas (CH4)? Two moles of air are needed for the constituents on both sides of the reaction, one C, four H, and four O, to be the same. Nothing is created or destroyed, only rearranged. For this purpose, N, for nitrogen in the air doesn’t matter. It doesn’t react with anything.
Why two moles of air per mole of natural gas (CH4)? Two moles of air are needed for the constituents on both sides of the reaction, one C, four H, and four O, to be the same. Nothing is created or destroyed, only rearranged. For this purpose, N, for nitrogen in the air doesn’t matter. It doesn’t react with anything.
Here is my proof of the 117 lb claim by EIA, courtesy of Craig Kroger, whose tool I used through ha school, college, and professionally to this day. We go from a million Btu to volume of natural gas to volume of CO2 to mass of CO2: 118 lbs. Victory!
 Methodology
Methodology
Start by drawing a horizontal line and then walk across, left to right, until you get what you’re after. All the units, e.g., Btu, mole, cf, cancel, leaving me with pounds.
The terms that are stacked in pairs are equals. For instance, 100 cf (or ft3) of natural gas has about 102,000 Btu. Gas meters measure cubic feet of consumption. Every mole of methane (CH4) results in 44 grams of CO2 (one carbon at 12 grams and two oxygens at 16 grams each). One kilogram, which equals 1000 grams, equals 2.2 lbs. Multiply the top; multiply the bottom. Divide. 118 lbs.
So, was that air you displace while standing on a scale negligible? Intuitive analysis (company value) tells me, just assume the body is 100% water, and for density purposes, that is close enough. We go from pounds of body weight to volume of air displaced by your body to grams per air volume displaced to lbs of air.
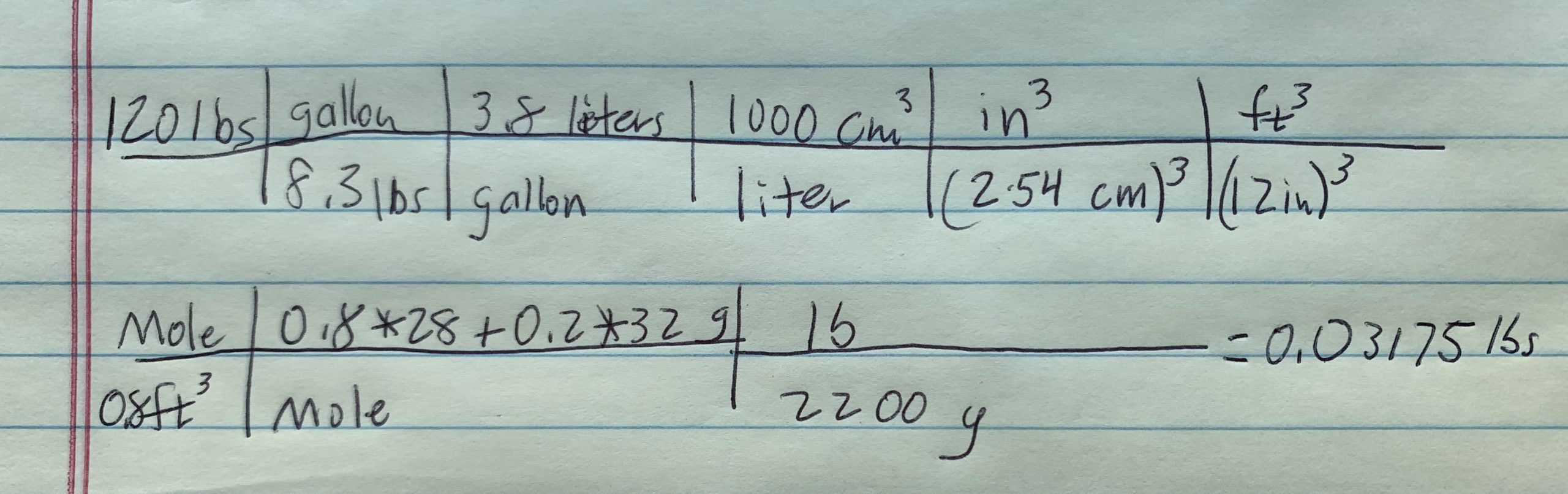 You might be thinking, “why use gallons”? Use whatever you can associate with a unit (like lbs to gallons) that gets you to the result. I like to use what’s in my brain rather than what’s on the innertube. It’s faster.
You might be thinking, “why use gallons”? Use whatever you can associate with a unit (like lbs to gallons) that gets you to the result. I like to use what’s in my brain rather than what’s on the innertube. It’s faster.
[1] Enough with the “polar vortex” bologna. This is a grind.
[2] Science, technology, engineering, and mathematics
[3] Anything with carbon and hydrogen in it: wood, paper, sugar, plastic, marshmallows, corn oil, coal, lard, etc.

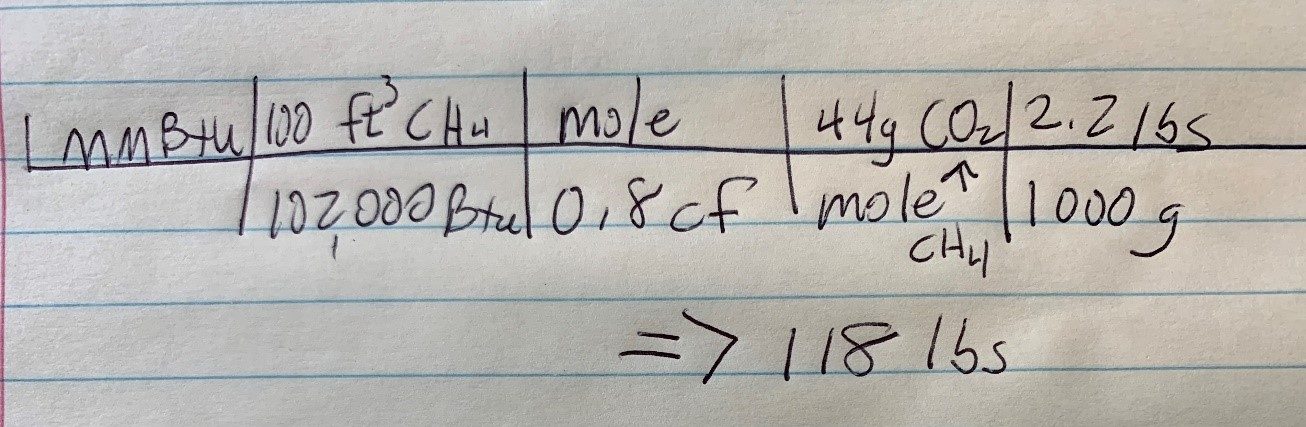 Methodology
Methodology